The Science Behind Math Mastery
The five strands of mathematical proficiency—productive disposition, conceptual understanding, procedural fluency, strategic competence, and adaptive reasoning—work together like strands of a rope, creating a strong foundation for mathematical success.
The five strands of mathematical proficiency.
Productive Disposition
Providing the Right Scaffolds
Productive Disposition means seeing it as sensible, useful, and doable. With frequent practice opportunities and automatic targeted scaffolding, students build confidence and self-efficacy.
The most effective scaffolding happens when the elements of a task are adjusted to enable independent completion by the student, rather than altering the task itself.
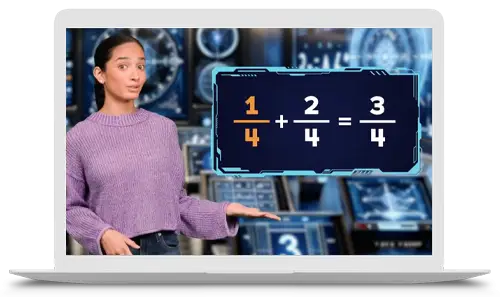
Conceptual Understanding
Deepening Math Connections
Understanding math concepts means knowing how and why things work, lessons are designed to build on what students already know, supporting longer-term retention and knowledge transfer.
Systematic instruction can help students connect a tool they are already familiar with, like a number line, to a new concept.
Procedural Fluency
Mastering Math Skills
Procedural fluency involves carrying out mathematical procedures accurately, efficiently, and flexibly. Adaptive learning games enhance procedural fluency by offering frequent practice, immediate feedback, and strategic thinking.
Modern, highly engaging gameplay to give students lots of practice with immediate feedback, making sure they master these skills in a fun way.
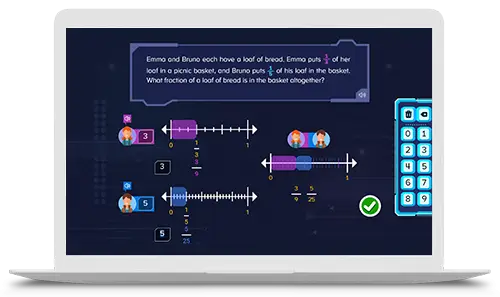
Strategic Competence
Enhancing Problem-Solving Abilities
Strategic Competence is the ability to thoughtfully formulate, represent, and solve mathematical problems. Meaningful word problems with real-world storylines can sustain student engagement.
In My Math Academy, a variety of problem-solving tasks are applied to real-world problems.
Adaptive Reasoning
Building Logical Thinking
Adaptive reasoning is about logical thought, reflection, explanation, and justification. Students can develop adaptive reasoning skills when they have regular opportunities to talk about the concepts and procedures they are using to justify their mathematical claims.
Small group and 1:1 mini-lessons that encourage students to have meaningful discussions about concepts and procedures.
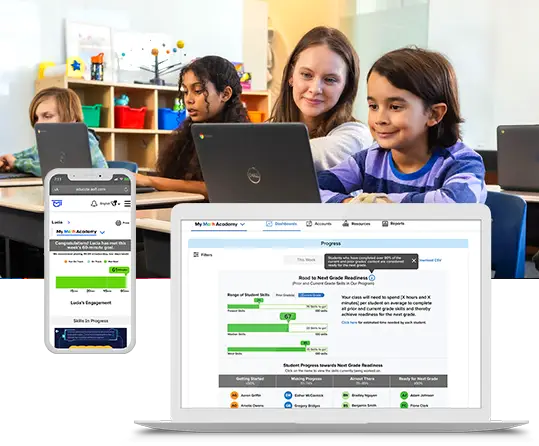
Resources
Building Logical Thinking
For more information on the five key areas of math proficiency, check out these resources: